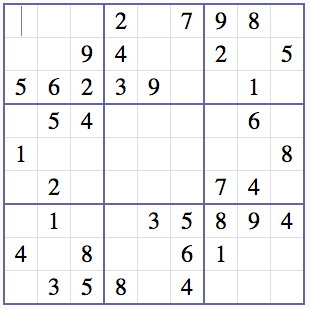
I have written a program to solve Sudoku puzzles as a (fun?) way to learn Python. It solves puzzles as a human would, using reasoning algorithms rather than brute force/backtracking, as I thought it would a more interesting challenge that way.
I really looking for any feedback on how to reduce indentation, lines of code, or increase efficiency. I'm sure the functions that I've repeated for columns/rows/subgrids can be merged into one, but I just can't think of an elegant way. Any general feedback would be more than welcome too, please lay into the code as much as possible. I'm a 2nd year computing (CS but lite) student if that makes any difference but probably not.
I appreciate this involves domain specific knowledge i.e. how to solve Sudoku puzzles. I created all the algorithms using only the following resource and all of the techniques contained in the program are described here for anyone interested.
import itertools
# TERMINOLOGY
# grid: The main 9x9 grid of the puzzle.
# sub-grid: A 3x3 sub-grid within the main grid.
# square: A single square within the grid.
# row: A whole row of squares within the main grid.
# column: A whole column of squares within the main grid.
# sub-row: A row of three squares in a sub-grid.
# sub-column: A column of three squares in a sub-grid.
# candidate: A possible solution for a square.
# solved: A square is solved when the correct value is filled.
#
# For in-depth descriptions of the various sudoku solving techniques
# used in this program, visit:
# http://www.paulspages.co.uk/sudoku/howtosolve/index.htm
# This is the sole resource used to generate the techniques
# found in this program.
# Holds puzzle itself as 2d list. Blank squares represented as 0.
# Individual square access: main_grid[x][y]
main_grid = [[] for x in range(0, 9)]
# Holds all possible candidates for each square as a 2d list of sets.
# Individual square set access: candidates_grid[x][y]
candidates_grid = [[set() for y in range(0, 9)] for x in range(0, 9)]
# Holds all solved values in an individual row/col/sub-grid
col_set = [set() for x in range(0, 9)] # Access: col_set[x]
row_set = [set() for x in range(0, 9)] # Access: row_set[y]
sub_grid_set = [[set() for y in range(0, 3)] for x in range(0, 3)]
# Misc sets used for solving techniques/optimisation
full_set = set({1, 2, 3, 4, 5, 6, 7, 8, 9})
coordinates_set = set({0, 1, 2, 3, 4, 5, 6, 7, 8})
# Populates main_grid, candidates_grid, row/col/block sets from data file
def init():
puzzle = open('../puzzles/extreme2.txt', 'r')
for y in range(0, 9):
next_line = puzzle.readline()
for x in range(0, 9):
main_grid[x].append(int(next_line[x]))
if next_line[x] != '0':
col_set[x].add(int(next_line[x]))
row_set[y].add(int(next_line[x]))
sub_grid_set[int(x / 3)][int(y / 3)].add(int(next_line[x]))
for y in range(0, 9):
for x in range(0, 9):
if main_grid[x][y] == 0:
candidatesSet = set.union(row_set[y], col_set[x],
sub_grid_set[int(x / 3)][int(y / 3)])
candidates_grid[x][y] = full_set.difference(candidatesSet)
def iter_over_subgrids(func, *args):
for sub_grid_y in range(0, 3):
for sub_grid_x in range(0, 3):
func(sub_grid_x, sub_grid_y, *args)
def iter_over_line(func, *args):
for square in range(0, 9):
func(square, *args)
def print_main_grid():
for y in range(0, 9):
for x in range(0, 9):
print(main_grid[x][y], end="")
if x % 3 == 2:
print(" ", end="")
print("")
def print_candidates_grid():
for y in range(0, 9):
for x in range(0, 9):
print(candidates_grid[x][y], " ", end="")
print("")
def is_solved():
for y in range(0, 9):
if len(row_set[y]) != 9:
return 0
return 1
# Writes solution to main_grid, updates sets and tables.
def pencil_in(solution, x, y, func):
sub_grid_x = int(x / 3)
sub_grid_y = int(y / 3)
main_grid[x][y] = solution
row_set[y].add(solution)
col_set[x].add(solution)
sub_grid_set[sub_grid_x][sub_grid_y].add(solution)
candidates_grid[x][y].clear()
for sg_y in range(sub_grid_y * 3, sub_grid_y * 3 + 3):
for sg_x in range(sub_grid_x * 3, sub_grid_x * 3 + 3):
candidates_grid[sg_x][sg_y].discard(solution)
for i in range(0, 9):
candidates_grid[x][i].discard(solution)
candidates_grid[i][y].discard(solution)
# Solves squares that have only one candidate.
def single_candidate_square(y):
for x in range(0, 9):
if len(candidates_grid[x][y]) == 1:
pencil_in(candidates_grid[x][y].pop(), x, y,
single_candidate_square)
# Solves squares where candidate appears only once in a row.
def single_sq_candidate_row(y):
for candidate in full_set.difference(row_set[y]): # Skip solved values
count = 0
prev_x = 0
for x in range(0, 9):
if candidate in candidates_grid[x][y]:
count += 1
prev_x = x
if count == 1:
pencil_in(candidate, prev_x, y, single_sq_candidate_row)
# As single_sq_candidate_row, for columns.
def single_sq_candidate_col(x):
for candidate in full_set.difference(col_set[x]): # Skip solved values
count = 0
prev_y = 0
for y in range(0, 9):
if candidate in candidates_grid[x][y]:
count += 1
prev_y = y
if count == 1:
pencil_in(candidate, x, prev_y, single_sq_candidate_col)
# As single_sq_candidate_row, for subgrids.
def single_sq_candidate_subgrid(sub_grid_x, sub_grid_y):
for candidate in full_set.difference(sub_grid_set[sub_grid_x][sub_grid_y]):
count = 0
prev_coords = [0, 0]
for y in range(sub_grid_y * 3, sub_grid_y * 3 + 3):
for x in range(sub_grid_x * 3, sub_grid_x * 3 + 3):
if candidate in candidates_grid[x][y]:
count += 1
prev_coords[0] = x
prev_coords[1] = y
if count == 1:
pencil_in(candidate, prev_coords[0], prev_coords[1],
single_sq_candidate_subgrid)
# Finds candidates in block that lie only on one subrow,
# removes candidates from rest of row.
def number_claiming_row(sub_grid_x, sub_grid_y):
# Get set of all candidates each subrow
subrow_sets = [set(), set(), set()]
for y in range(sub_grid_y * 3, sub_grid_y * 3 + 3):
for x in range(sub_grid_x * 3, sub_grid_x * 3 + 3):
subrow_sets[y % 3] = subrow_sets[y % 3].union(candidates_grid[x][y])
# Get candidates which only appear in one subrow
claimed = [subrow_sets[0].difference(subrow_sets[1], subrow_sets[2])]
claimed.append(subrow_sets[1].difference(subrow_sets[0], subrow_sets[2]))
claimed.append(subrow_sets[2].difference(subrow_sets[0], subrow_sets[1]))
# Remove candidates from other subrows in parent row
for sub_row in range(0, 3):
for claimant in set(claimed[sub_row]):
for x in range(0, 9):
if int(x / 3) != sub_grid_x:
candidates_grid[x][sub_grid_y * 3 + sub_row].discard(claimant)
# As number_claiming_row, but for columns
def number_claiming_col(sub_grid_x, sub_grid_y):
# Get set of all candidates each subcolumn
subcol_sets = [set(), set(), set()]
for x in range(sub_grid_x * 3, sub_grid_x * 3 + 3):
for y in range(sub_grid_y * 3, sub_grid_y * 3 + 3):
subcol_sets[x % 3] = subcol_sets[x % 3].union(candidates_grid[x][y])
# Get candidates which only appear in one subcolumn
claimed = [subcol_sets[0].difference(subcol_sets[1], subcol_sets[2])]
claimed.append(subcol_sets[1].difference(subcol_sets[0], subcol_sets[2]))
claimed.append(subcol_sets[2].difference(subcol_sets[0], subcol_sets[1]))
# Remove candidates from other subcolumns in parent column
for sub_col in range(0, 3):
for claimant in set(claimed[sub_col]):
for y in range(0, 9):
if int(y / 3) != sub_grid_y:
candidates_grid[sub_grid_x * 3 + sub_col][y].discard(claimant)
# Finds sets of n squares in a row where:
# - No squares contain more than n candidates each.
# - The cardinality of the set of all the candidates in squares is n.
# All candidates in that set can be assumed to lie in those squares,
# so the set of candidates can be removed from all other squares in
# that row. Sudoku solvers may already know disjoint subsets as "pairs"
# or "triples".
#
# Basic example: three squares in a row contain the candidate sets
# {2,4}, {2,7} and {4,7} respectively. All three squares contain no
# more than three candidates, and the set of all candidates is {2,4,7},
# which has a cardinality of three. It can then be assumed that those
# squares MUST contain 2, 4 and 7 and nothing else. Any squares outside
# those three in the row can then have the candidates 2, 4 and 7 removed.
def disjoint_subsets_row(y, n):
sets = []
# Get all candidate sets in row with cardinality no greater than n
for x in range(0, 9):
if 1 < len(candidates_grid[x][y]) <= n:
sets.append(candidates_grid[x][y])
# For all disjoint subsets found, remove candidates from other squares
for d in get_disjoint_subsets(sets, n):
for x in range(0, 9):
if not candidates_grid[x][y].issubset(d):
candidates_grid[x][y] = candidates_grid[x][y].difference(d)
# As disjoint_subsets_row, for columns
def disjoint_subsets_col(x, n):
sets = []
# Get all candidate sets in row with cardinality no greater than n
for y in range(0, 9):
if 1 < len(candidates_grid[x][y]) <= n:
sets.append(candidates_grid[x][y])
# For all disjoint subsets found, remove candidates from other squares
for d in get_disjoint_subsets(sets, n):
for y in range(0, 9):
if not candidates_grid[x][y].issubset(d):
candidates_grid[x][y] = candidates_grid[x][y].difference(d)
# As disjoint_subsets_row, for sub-grids.
def disjoint_subsets_subgrid(sub_grid_x, sub_grid_y, n):
sets = []
# Get all candidate sets in row with cardinality no greater than n
for y in range(sub_grid_y * 3, sub_grid_y * 3 + 3):
for x in range(sub_grid_x * 3, sub_grid_x * 3 + 3):
if 1 < len(candidates_grid[x][y]) <= n:
sets.append(candidates_grid[x][y])
# For all disjoint subsets found, remove candidates from other squares
for d in get_disjoint_subsets(sets, n):
for y in range(sub_grid_y * 3, sub_grid_y * 3 + 3):
for x in range(sub_grid_x * 3, sub_grid_x * 3 + 3):
if not candidates_grid[x][y].issubset(d):
candidates_grid[x][y] = candidates_grid[x][y].difference(d)
def get_disjoint_subsets(sets, n):
disjoint_subsets = set()
# For each combination of n sets in sets
for combination in itertools.combinations(sets, n):
superset = set()
# For each individual set in combination
for c in combination:
superset = superset.union(c)
if len(superset) == n:
# Cardinality of candidate superset in combination is n, is djss.
disjoint_subsets.add(frozenset(superset))
return disjoint_subsets
# Runs through solving techniques until puzzle solved or no possible solution.
def solve():
for x in range(0, 100):
iter_over_line(single_candidate_square)
iter_over_line(single_sq_candidate_row)
iter_over_line(single_sq_candidate_col)
iter_over_subgrids(single_sq_candidate_subgrid)
iter_over_subgrids(number_claiming_row)
iter_over_subgrids(number_claiming_col)
for n in range(2, 5):
iter_over_line(disjoint_subsets_row, n)
iter_over_line(disjoint_subsets_col, n)
iter_over_subgrids(disjoint_subsets_subgrid, n)
if is_solved() == 1:
print_main_grid()
break
init()
solve()
Puzzles are solved in the text file in the format:
400700900
000004000
070280006
500000010
301060408
020000009
900036020
000800000
004001003
Example of a Sudoku game:
4 - - | 7 - - | 9 - -
- - - | - - 4 | - - -
- 7 - | 2 8 - | - - 6
------|-------|------
5 - - | - - - | - 1 -
3 - 1 | - 6 - | 4 - 8
- 2 - | - - - | - - 9
------|-------|------
9 - - | - 3 6 | - 2 -
- - - | 8 - - | - - -
- - 4 | - - 1 | - - 3
The above is an "extreme" level puzzle.