Follow up on Plotting polynomial roots
I rewrote the program to C++ and am now using GNU GSL scientific library and gnuplot and also used OpenMP for multithreading. The result is that it takes about 7 seconds compared to 14 hours when I used python with numpy and matplotlib.
I added the functionality to plot the image with different colours based on the density of the points with a radius of 0.1. The algorithm for this is O(n^2) which is very slow. I basically brute force to check the distance for each point to every other point in the system and check if it is <= 0.1 and if it is I add to the neighbour count for that point. In the end I map the neighbour count to a scalar between 0 and 1.5 which represents the colour.
#include <stdlib.h>
#include <stdio.h>
#include <string.h>
#include <gsl/gsl_poly.h>
#include <cmath>
#define maxd(a,b) (((a) > (b)) ? (a) : (b))
#define mind(a,b) (((a) < (b)) ? (a) : (b))
unsigned long long cur_root;
unsigned long long capacity;
void
polynomial_recursive(double **roots, double min_x, double max_x, int min_degree, int max_degree, int cur_degree, double *coeff)
{
if (cur_degree <= max_degree)
{
for (double c = -4; c <= 4; c++)
{
double *new_coeff;
new_coeff = (double *) malloc (sizeof (double) * (cur_degree + 1));
if (new_coeff == NULL)
{
perror ("malloc failed");
}
memcpy (new_coeff, coeff, cur_degree * sizeof (double));
new_coeff[cur_degree] = c;
if (cur_degree >= min_degree && new_coeff[cur_degree] != 0)
{
double z[cur_degree*2];
gsl_poly_complex_workspace *w
= gsl_poly_complex_workspace_alloc (cur_degree + 1);
gsl_poly_complex_solve (new_coeff, cur_degree + 1, w, z);
gsl_poly_complex_workspace_free (w);
for (int i = 0; i < cur_degree; i++)
{
if (cur_root + 2 >= capacity)
{
capacity *= 2;
double *tmp = (double *) realloc (*roots, capacity * sizeof (double));
if (tmp == NULL)
{
perror ("realloc failed");
}
else
{
*roots = tmp;
}
}
(*roots) [cur_root++] = z[2*i];
(*roots) [cur_root++] = z[2*i+1];
}
}
#pragma omp task shared(new_coeff)
polynomial_recursive (roots, min_x, max_x, min_degree, max_degree, cur_degree + 1, new_coeff);
#pragma omp taskwait
free (new_coeff);
}
}
}
unsigned long long getDensityForPoint(double x, double y, double *points, int size, double radius)
{
unsigned long long count = 0;
for (int i = 0; i < size; i += 2)
{
double x2 = points[i];
double y2 = points[i+1];
if (x != x2 || y != y2)
{
double dist = fabs(x2 - x) + fabs(y2 - y);;
if (dist <= radius)
{
count++;
}
}
}
return count;
}
double
calculateDensityScalarFromDensity(unsigned long min, unsigned long max, unsigned long density)
{
return 0.0 + ((1.5 - 0.0) / (double)(max - min)) * (density - min);
}
int
main(void)
{
double *roots;
unsigned long long *densities, min, max;
capacity = 100;
cur_root = 0;
roots = (double *) malloc (capacity * sizeof (double));
if (roots == NULL)
{
perror ("malloc failed");
}
#pragma omp parallel
{
#pragma omp single
{
polynomial_recursive (&roots, -4, 4, 2, 5, 0, NULL);
}
}
densities = (unsigned long long *) malloc (cur_root / 2 * sizeof (unsigned long long));
max = 0;
min = ~0;
#pragma omp parallel for
for (int i = 0; i < cur_root; i += 2)
{
densities[i / 2] = getDensityForPoint(roots[i], roots[i+1], roots, cur_root, 0.1);
max = maxd(densities[i / 2], max);
min = mind(densities[i / 2], min);
}
printf("max %llu, min %llu\n", max, min);
FILE *gnuplotPipe = popen ("gnuplot -persistent", "w");
FILE *temp = fopen("data.temp", "w");
#pragma omp parallel for
for (int i = 0; i < cur_root; i += 2)
{
fprintf (temp, "%lf %lf %lf\n", roots[i], roots[i+1], calculateDensityScalarFromDensity(min, max, densities[i / 2]));
}
free(densities);
free(roots);
fprintf (gnuplotPipe, "set zrange [0.0:1.5]\nset cbrange [0.0:1.5]\nset term pngcairo\nset terminal png size 1920,1080\nset output \"plot.png\"\nset palette defined (0 \"black\", 0.5 \"red\", 1 \"yellow\", 1.5 \"white\")\nplot 'data.temp' with points pointtype 5 pointsize 0.001 palette\n");
}
It is this part that is taking so long:
for (int i = 0; i < cur_root; i += 2)
{
densities[i / 2] = getDensityForPoint(roots[i], roots[i+1], roots, cur_root, 0.1);
max = maxd(densities[i / 2], max);
min = mind(densities[i / 2], min);
}
I am using the manhattan distance because that is the fastest I could think of. The problem is that I'm doing this for very complex polynomials. I set min_degree to 20 and max_degree to 22 and instead of using between -4 and 4 I use only either -PI or +PI so nothing in between.
Going up to polynomials of 22 order and only using two different coefficients I calculated that there would be about 300 million roots and so with a O(n^2) time complexity it would take around 75 days to complete which is insane. I've heard about k-d trees but haven't successfully implemented them to gain any major speed advantage. Another idea I had is to downscale the data.
How do I speed up the density calculation?
Here is another image that I generated with max_degree 5 and coefficients between -4 and 4 and the density function enabled (black is low density, white is high density):

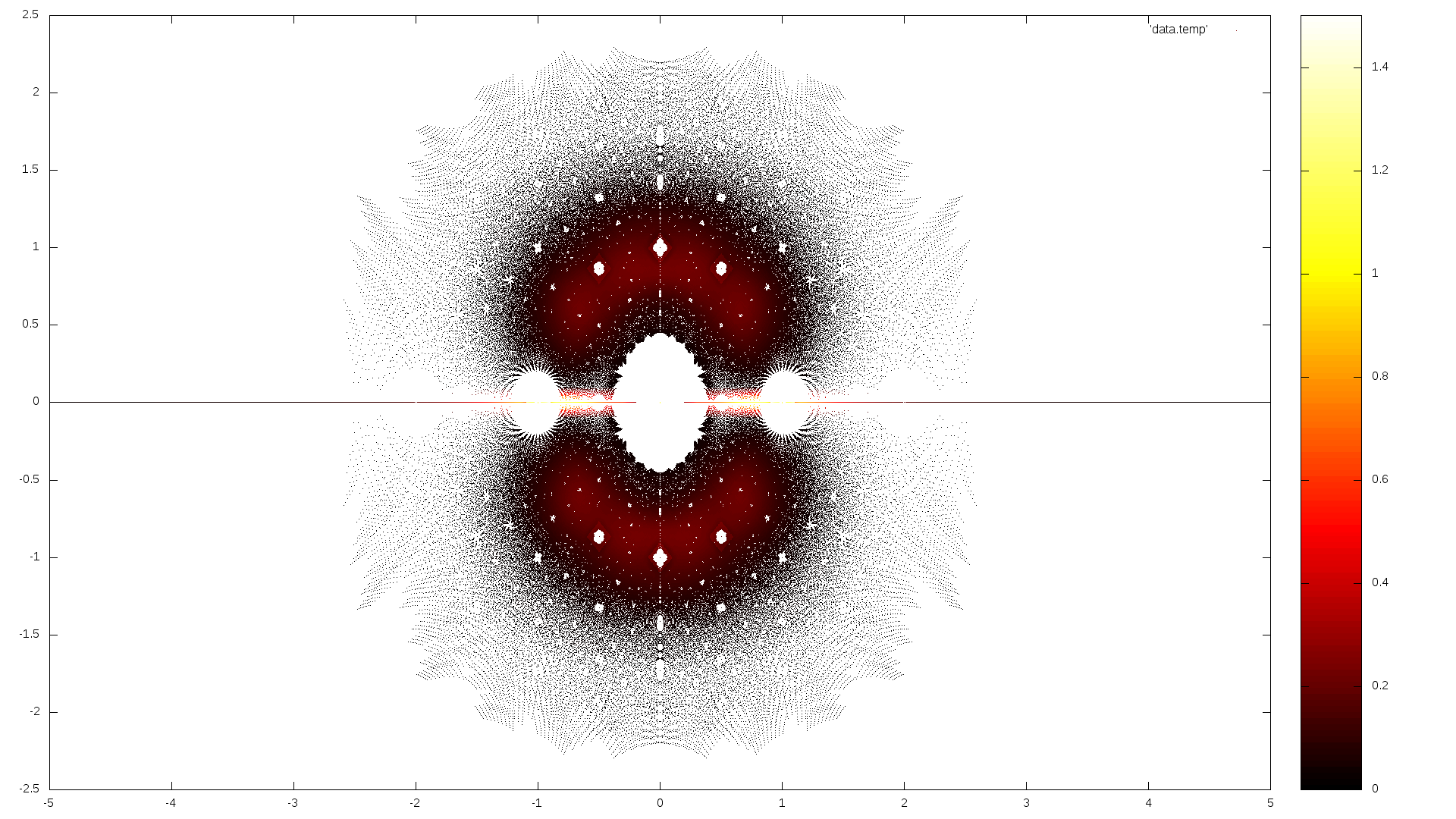
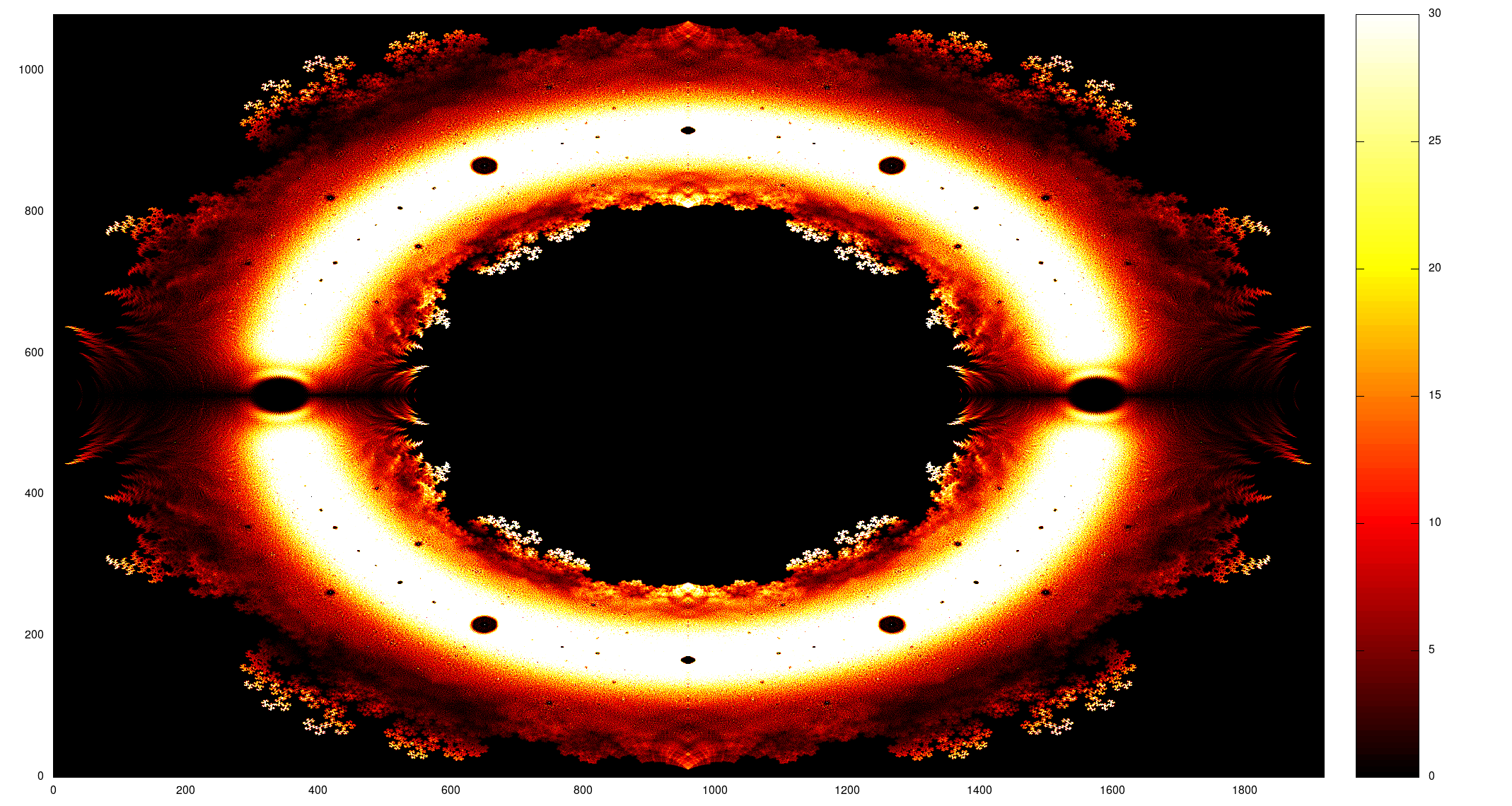
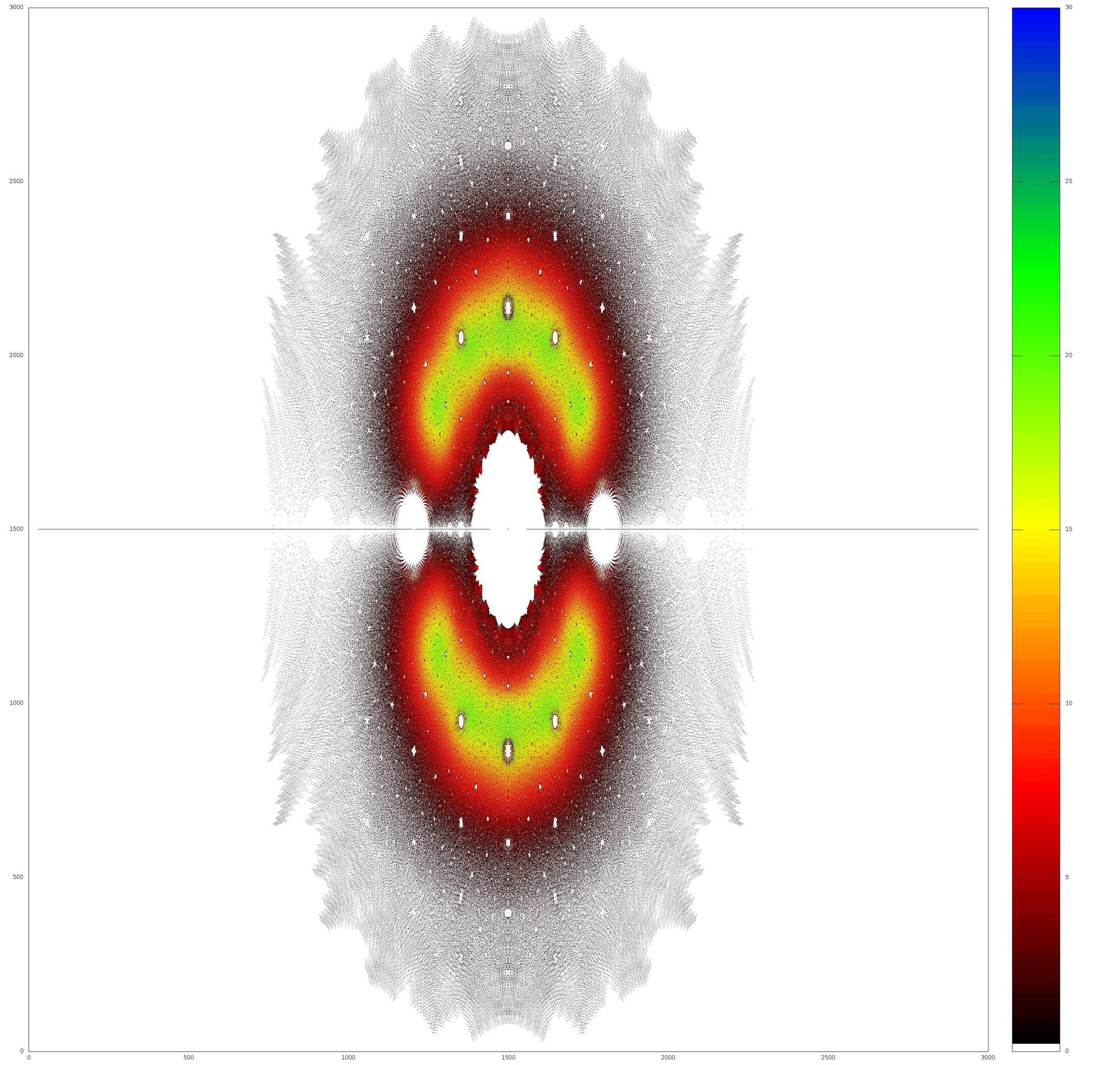
#pragma omp parallel forhint to parallelize this loop? (BTW, parallelizing a for loop that callsfprintfis somewhere between silly and a bad idea…). \$\endgroup\$