An L-system is a rewriting system that can be used to generate fractals and space filling curves, because of its recursive nature.
Some L-systems for mathematical curves can be found here.
An example L-system:
Axiom:
$$FX+FX+$$
Production rules:
$$X → X+YF$$ $$Y → FX-Y$$
Angle: $$\theta = 90$$
In this example, F means "move forward", "+" means "turn right by angle theta, and "-" means "turn left by angle theta". These are simple commands that are followed by a "turtle", which moves around and creates a path. The letters X and Y are not commands, they are only used for the substitution process.
The axiom states "move forward, turn right, move forwards, turn right". After applying the production rules, the command string becomes
$$FX+YF+FX+YF+$$
Performing many iterations produces long, complicated chains of commands. As the turtle follows the commands, very interesting shapes can be produced.
import matplotlib.pyplot as plt
import numpy as np
from math import sin, cos, atan2, radians
class turtle:
"""
A turtle is a simple object with a direction and a position.
It can follow two basic commands: move forward and turn by an angle
"""
def __init__(self):
self._direction = np.array([1, 0]) # 2D direction vector
self._position = np.array([0, 0]) # 2D position vector
def forward(self):
"""
Move turtle forward by one unit.
"""
pos = self._position
dirn = self._direction
self._position = np.add(pos, dirn)
def rotate(self, theta):
"""
Rotate turtle direction by angle theta in degrees.
"""
(x, y) = self._direction
current_angle = atan2(y, x)
new_angle = current_angle + radians(theta)
self._direction = [cos(new_angle), sin(new_angle)]
def L_system(commands, axiom, production_rules, theta, n_iterations):
"""
Executes the commands of an L-system on a turtle object,
and returns the resulting positions.
Beginning with a string of simple commands, this string is made longer by
replacing single characters with longer strings, in a recursive manner.
By completing a number of iterations of this process, a long command string
is generated. A 'turtle' object then follows these commands in order.
It can only move forward or change its direction. The positions of the turtle are
returned in a matrix.
Parameters
----------
commands : dict
Maps single characters to function calls written as strings
The functions are performed on a turtle object
e.g. {'+': 't.rotate(-theta)', '-': 't.rotate(theta)', 'F': 't.forward()'}
axiom : str
The initial string of command characters.
The associated function calls of these characters are found in param commands
e.g. 'FX+FX+'
production_rules : dict
Maps single character strings to more complicated strings of characters
The value strings replace the key strings on each new iteration
e.g. {'X': 'X+YF', 'Y': 'FX-Y'}
theta : int
Angle of rotation, in degrees
e.g. 90
n_iterations : int
Number of iterations for the L system
e.g. 5
Returns
-------
positions : numpy matrix
The positions of the turtle, while following commands in the final command string
"""
command_string = axiom # Begin commands with only the axiom
for iteration in range(n_iterations):
new_command_string = str()
for char in command_string:
if char in production_rules:
new_command_string += production_rules[char]
else:
new_command_string += char
command_string = new_command_string
n_commands = len(command_string) # Total number of commands for the turtle
t = turtle() # Initialize a turtle at position [0, 0]
positions = np.zeros((n_commands, 2))
for i, command in enumerate(command_string):
if command in commands:
exec(commands[command]) # Perform command on turtle
positions[i, :] = t._position
return positions
commands = {
'F': 't.forward()',
'+': 't.rotate(-theta)',
'-': 't.rotate(theta)',
}
axiom = 'FX+FX+'
production_rules = {
'X': 'X+YF',
'Y': 'FX-Y'
}
n_iterations = 11
theta = 90
positions = L_system(commands, axiom, production_rules, theta, n_iterations)
positions = L_system(commands, axiom, production_rules, theta, n_iterations)
plt.plot(positions[:, 0], positions[:, 1])
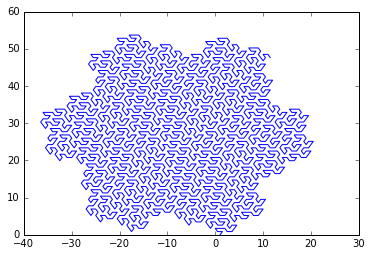
The output of this code is the Twin Dragon fractal:
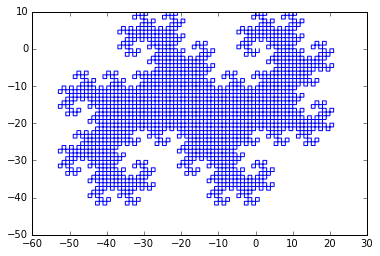
Other examples include the Sierpinski Arrowhead curve:
axiom = 'FX'
production_rules = {
'X': 'YF+XF+Y',
'Y': 'XF-YF-X'
}
n_iterations = 8
theta = 60
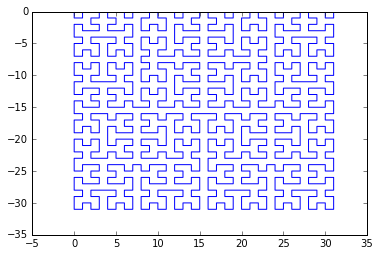
The Hilbert curve:
axiom = 'X'
production_rules = {
'X': '+YF-XFX-FY+',
'Y': '-XF+YFY+FX-'
}
n_iterations = 5
theta = 90
And the Gosper Curve:
commands = {
'A': 't.forward()',
'B': 't.forward()',
'+': 't.rotate(-theta)',
'-': 't.rotate(theta)',
}
axiom = 'A'
production_rules = {
'A': 'A-B--B+A++AA+B-',
'B': '+A-BB--B-A++A+B'
}
n_iterations = 4
theta = 60