The script below is used to determine relay operating times, as defined in the standard IEC 60255. The equation and relevant information can be found here.
curve_equation takes three input values, and outputs a list of values representing the trip times for currents in the fixed range np.logspace(np.log10(Is), 5, 1e2).
The part in the bottom plots the trip times for the different curves. The function curve_equation will be used to much more than that, so I'm mostly interested in improvements to that one.
# -*- coding: utf-8 -*-
"""
Created on Sun Apr 29 13:28:26 2018
@author: Stewie
"""
import numpy as np
import matplotlib.pyplot as plt
def curve_equation(curve_name, T, Is):
"""
This function calculates and returns the inverse curves for
the selected curve type.
Input:
curve_name: one of the strings: "SIT", "VIT", "LTI", "EIT", "UIT"
T: The time dial setting. Typically in the range 0.1 ... 1
Is: The current setting. Typically in the range 100-10000
The appropriate k, alpha and theta values are selected based on the
curve type.
"""
# Define constants to be used in the curve equation
k = (0.14, 13.5, 120, 80, 315.2)
alpha = (0.02, 1, 2, 2, 2.5)
beta = (2.97, 1.5, 13.33, 0.808, 1)
curve_types = ("SIT", "VIT", "LTI", "EIT", "UIT")
# idx is the index of the values we want
try:
idx = curve_types.index(curve_name)
except:
print("Non-existing curve-type")
return
I = np.logspace(np.log10(Is), 5, 1e2)
def td_equation():
td = k[idx]/((I/Is)**alpha[idx]-1) * (T / beta[idx])
return(td)
return(td_equation())
# For testing / plotting purposes:
curve_types = ("SIT", "VIT", "LTI", "EIT", "UIT")
I = np.logspace(1, 5, 1e2)
for curve_type in curve_types:
plt.loglog(I, curve_equation(curve_type, 1, 1e2), label=curve_type)
plt.xlabel("Current [A]")
plt.ylabel("Time [s]")
plt.title("IEC Time/current curves")
plt.grid()
plt.legend()

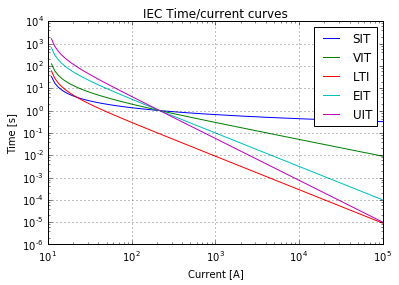
NameError: name 'I' is not definederror on theplt.loglog(I, …)line. \$\endgroup\$Iwas already stored as a variable outside the function so I didn't get an error message when I removed it. \$\endgroup\$