Introduction

This is yet another data structure I'm going over again for the algorithms course. This time it is binary search tree.

Implemented operations:

• Insert

• Exists

• Remove

• Clear

• Move constructor and assignment, destructor

There are some tests for remove function below the data structure itself. I tested other functions, but the tests got overridden in the interim. They did pass though. I believe automated ones are not possible until I make the tree somehow traversable, but that's adventure for another time.

Concerns

• Extreme code duplication

The 4 case functions (nullptr, equal, less, greater) share the same control flow statements. I believe abstracting that away would make it worse though.

• Extremely complicated remove function

This one took around an hour of my time to get correct, from starting to write it to debugging the three cases I found and tested for.

• Overall just feels bad

It just gives that feeling. Or may be most of the algorithms I've seen are much more elegant than this.

Code

#include <stdexcept>
#include <type_traits>
#include <ostream>
#include <utility>

template <typename ValueType>
class binary_search_tree
{
    struct node
    {
        const ValueType value;
        node* left;
        node* right;
    };

    enum class direction
    {
        is_root,
        left,
        right
    };

    struct search_result
    {
        node* parent;
        node* target_child;
        direction parent_to_child;
    };

    node* root;
public:
    binary_search_tree() :
            root(nullptr)
    {}

    binary_search_tree(const binary_search_tree& other) = delete;
    binary_search_tree& operator=(const binary_search_tree& other) = delete;

    binary_search_tree(binary_search_tree&& other) :
            root(std::exchange(other.root, nullptr))
    {}

    binary_search_tree& operator=(binary_search_tree&& other) noexcept
    {
        std::swap(root, other.root);
        return *this;
    }

    bool try_insert(const ValueType& value)
    {
        return try_insert_helper(value, root);
    }

    bool exists(const ValueType& value)
    {
        return find_node(value, nullptr, root, direction::is_root).target_child != nullptr;
    }

    bool delete_if_exists(const ValueType& value)
    {
        auto [parent_node, node_with_value, parent_to_child] =
                find_node(value, nullptr, root, direction::is_root);

        if (node_with_value == nullptr)
            return false;

        if (node_with_value->left == nullptr)
        {
            auto old = node_with_value;
            switch (parent_to_child)
            {
                case direction::left:
                    parent_node->left = node_with_value->left;
                    break;
                case direction::right:
                    parent_node->right = node_with_value->right;
                    break;
                case direction::is_root:
                    root = root->right;
            }
            delete old;
            return true;
        }

        if (node_with_value->left->right == nullptr)
        {
            switch (parent_to_child)
            {
                case direction::left:
                    parent_node->left = node_with_value->right;
                    node_with_value->right->left = node_with_value->left;
                    break;
                case direction::right:
                    parent_node->right = node_with_value->right;
                    node_with_value->right->left = node_with_value->left;
                    break;
                case direction::is_root:
                    root->left->right = root->right;
                    root = root->left;
            }
            delete node_with_value;
            return true;
        }

        auto [suitable_parent, suitable_node] =
                find_suitable_node(node_with_value->left->right, node_with_value->left);
        switch (parent_to_child)
        {
            case direction::left:
                parent_node->left = suitable_node;
                suitable_node->right = node_with_value->right;
                suitable_node->left = node_with_value->left;
                break;
            case direction::right:
                parent_node->right = suitable_node;
                suitable_node->right = node_with_value->right;
                suitable_node->left = node_with_value->left;

                break;
            case direction::is_root:
                suitable_node->right = root->right;
                suitable_node->left = root->left;
                root = suitable_node;
        }
        suitable_parent->right = nullptr;
        delete node_with_value;

        return true;
    }

    void clear()
    {
        clear_helper(root);
    }

    void inorder_print(std::ostream& os)
    {
        if (root == nullptr)
            return;

        inorder_print_helper(os, root);
    }

    ~binary_search_tree()
    {
        clear();
    }

private:
    std::pair<node*, node*> find_suitable_node(node* start_position, node* parent)
    {
        if (start_position->right == nullptr)
            return {parent, start_position};
        return find_suitable_node(start_position->right, start_position);
    }

    void clear_helper(node* start_position)
    {
        if (start_position == nullptr)
            return;
        clear_helper(start_position->left);
        clear_helper(start_position->right);

        delete start_position;
    }

    search_result find_node(const ValueType& value,
                            node* parent,
                            node* current_node,
                            direction parent_to_child)
    {
        if (current_node == nullptr)
            return {nullptr, nullptr, direction::is_root};

        if (current_node->value == value)
            return {parent, current_node, parent_to_child};

        if (value < current_node->value)
            return find_node(value, current_node, current_node->left, direction::left);
        else
            return find_node(value, current_node, current_node->right, direction::right);
    }

    bool exists_helper(const ValueType& value,
                       node* current_node)
    {
        if (current_node == nullptr)
            return false;
        if (current_node->value == value)
            return true;

        if (value < current_node->value)
            return exists_helper(value, current_node->left);
        else
            return exists_helper(value, current_node->right);
    }

    void inorder_print_helper(std::ostream& os,
                              node*& current_node)
    {
        if (current_node == nullptr)
            return;

        inorder_print_helper(os, current_node->left);
        os << current_node->value << ' ';
        inorder_print_helper(os, current_node->right);
    }

    bool try_insert_helper(const ValueType& value,
                           node*& current_node)
    {
        if (current_node == nullptr)
        {
            current_node = new node{value};
            return true;
        }

        if (current_node->value == value)
            return false;

        if (current_node->value > value)
            return try_insert_helper(value, current_node->left);
        else
            return try_insert_helper(value, current_node->right);
    }
};

#include <iostream>
#include <sstream>

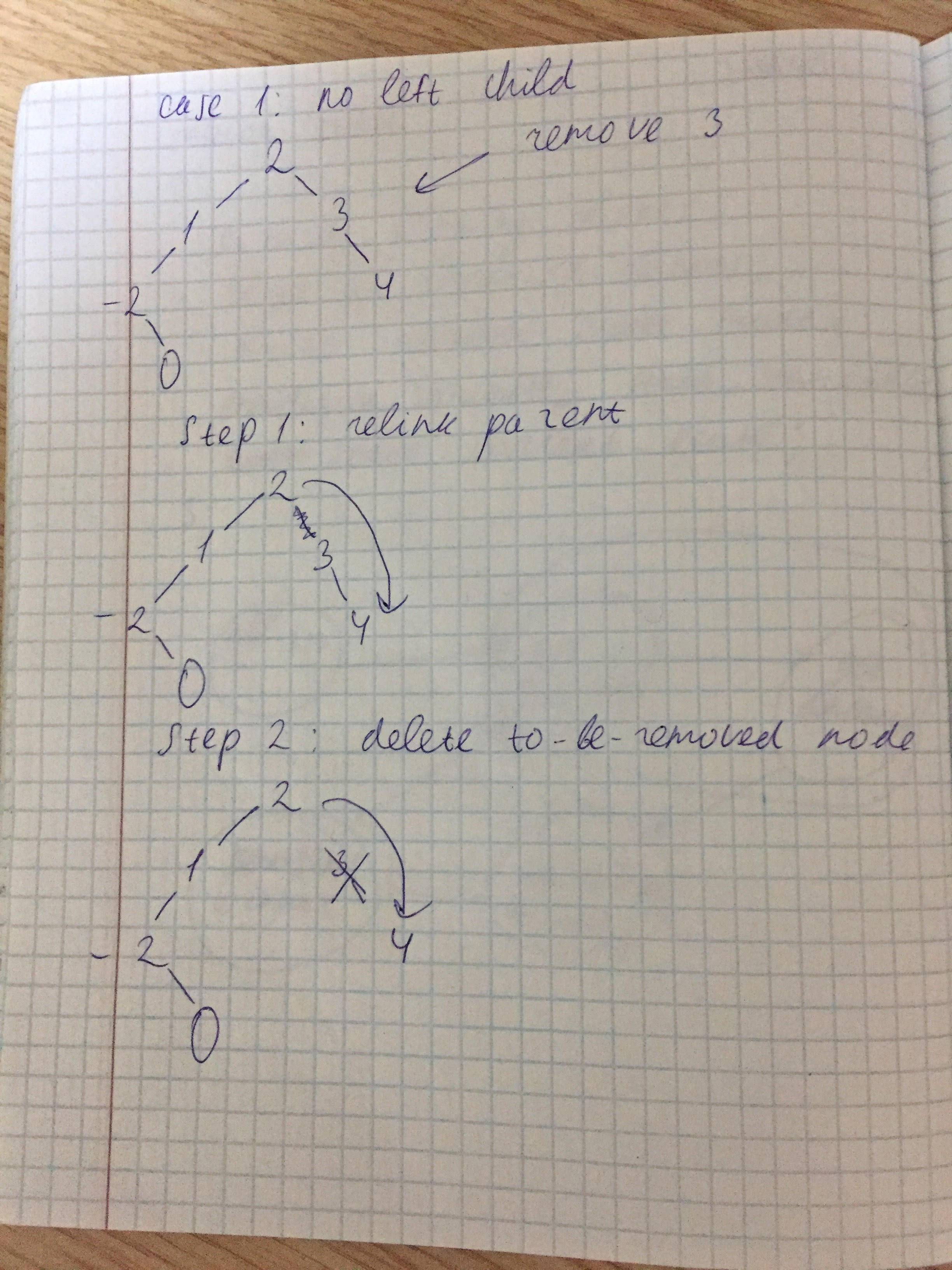
void test_remove_case_one()
{
    binary_search_tree<int> tree;
    tree.try_insert(2);
    tree.try_insert(3);
    tree.try_insert(1);
    tree.try_insert(4);
    tree.try_insert(-2);
    tree.try_insert(0);

    tree.delete_if_exists(3);
    std::ostringstream oss;
    tree.inorder_print(oss);
    if (oss.str() != "-2 0 1 2 4 ")
        throw std::logic_error("remove case one fails");
}

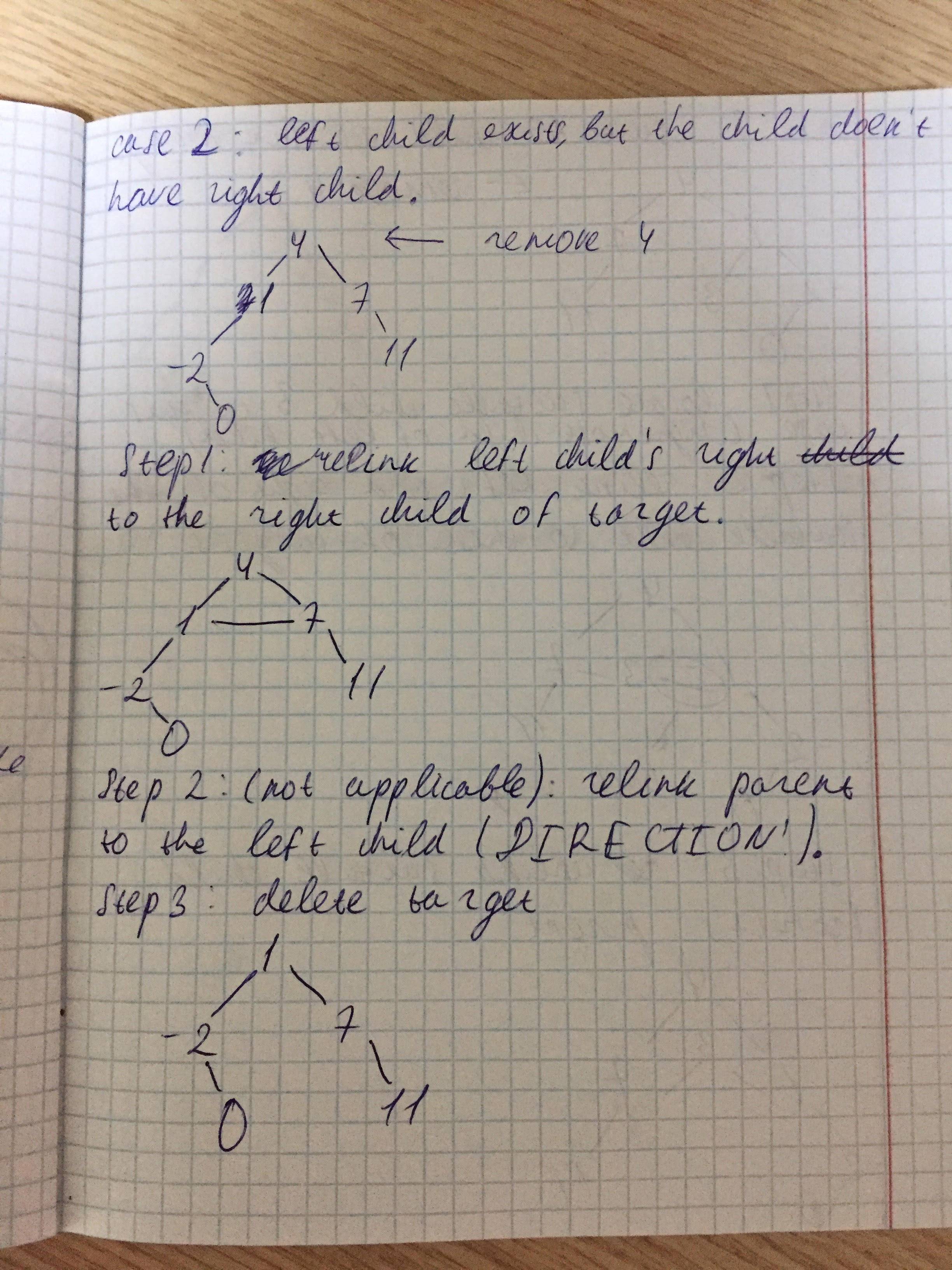
void test_remove_case_two()
{
    binary_search_tree<int> tree;
    tree.try_insert(4);
    tree.try_insert(7);
    tree.try_insert(11);
    tree.try_insert(1);
    tree.try_insert(-2);
    tree.try_insert(0);

    tree.delete_if_exists(4);
    std::ostringstream oss;
    tree.inorder_print(oss);
    if (oss.str() != "-2 0 1 7 11 ")
        throw std::logic_error("remove case two fails");
}

//almost like case 2, but has three added in it
void test_remove_case_three()
{
    binary_search_tree<int> tree;
    tree.try_insert(4);
    tree.try_insert(7);
    tree.try_insert(11);
    tree.try_insert(1);
    tree.try_insert(-2);
    tree.try_insert(0);
    tree.try_insert(3);

    tree.delete_if_exists(4);
    std::ostringstream oss;
    tree.inorder_print(oss);
    if (oss.str() != "-2 0 1 3 7 11 ")
        throw std::logic_error("remove case two fails");
}

int main(){
    std::cout << "running remove case 1...\n";
    test_remove_case_one();
    std::cout << "remove case 1 passed successfuly\n";
    std::cout << "running remove case 2...\n";
    test_remove_case_two();
    std::cout << "remove case 2 passed successfuly\n";
    std::cout << "running remove case 3...\n";
    test_remove_case_three();
    std::cout << "remove case 3 passed successfuly\n";
}

Explanations

I believe the implementation guidelines are very important, so I decided to keep them here, as the safest place to keep notes for me is SE posts (I know it is quite weird).

insert

Quite easy. Launch a recursion. If the current node is nullptr, insert at this node and return true (keep in mind that all pointers are passed by reference, thus the change will be reflected in the data structure itself. Also they always exist, no dangling references). If the value to-be-inserted is less than value in the node (IN THIS ORDER!), search right location to insert in the left subtree. If greater, search the location in the right subtree. If the value is equal, then return false.

exists

Almost the same like insertion, but the true/false cases are reversed. If value is equal to the value in the node, return true. If node is nullptr, return true (nowhere else to search).

remove

While searching for the node-to-remove, return these important three values: parent node of the target node, target node itself, the direction from parent to target child. If the value is in the tree, there are 3 cases (others are covered by these):

• to-be-removed node doesn't have left child (doesn't have suitable child)

Easy case. Relink parent of the to-be-removed node to the right child of the to-be-removed node (keep in mind DIRECTION!). Delete the to-be-removed node. Don't forget to update root if the to-be-removed node is root.

• to-be-removed node has left child, but the child doesn't have right child (has suitable child which is left child of to-be-removed node)

Somewhat easy too. Relink parent to the suitable child, change the right child of suitable child to the right child of to-be-removed node. Delete to-be-removed node. Update root if it is affected.

• to-be-removed node has suitable child which is far (> 1 depth) away

Find the rightmost child of the left child of to-be-removed node. Make sure the parent of the suitable child is no longer linked to the suitable child. Relink parent of the to-be-removed node to the suitable child. Relink left and right of the to-be-removed node the left and right of the suitable child, respectively. Delete to-be-removed node.

clear

If current node is nullptr, return. Go to the left, then to the right. Finally delete the current node.